g = gravitational field strength (= 9.8 N/kg)
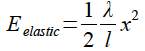
l = unstretched length,
x = extension (= current length - l )
Ework(= F.s = Fs cosθ)
Energy Methods
| kinetic energy |
Ekinetic = ½mv² |
m = mass, v = velocity |
| (gravitational) potential energy (change) |
Egravitational = mgΔh | m = mass, h = height (change), g = gravitational field strength (= 9.8 N/kg) |
| elastic (potential) energy |
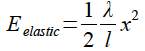 |
λ = modulus of elasticity, l = unstretched length, x = extension (= current length - l ) |
| work done against a force (often resistive or frictional, but not always) |
Ework = Fs Ework(= F.s = Fs cosθ) |
F = force, s = distance moved, using the component along the line of the force. The the vector formulation is not often needed. |
| power |
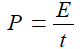 |
E can stand for any form of energy |
| power | P = F v |
F = force, P = power, v = velocity |
| ΔKE + ΔGPE + Δwork = 0 | The signs are all positive at this stage because this is just the standard equation |
| (½mv² - 2.5m) - 1960 m + 490 m = 0 |
Signs have become negative where our logic has told us that the value we are substituting is negative |
| ½v² = 1472.5 |
Cancelling by m and re-arranging |
| v = 54 m/s |
This is quite fast - about 100 mph. |